They're mixed up on the sheets, and there are FOUR total problems that start with the "squiggly" boxes, but I didn't tell them that first. I said they should look for 2 cards that pair up in such a way that they are ONE step away from each other either by an algebra law or by an identity. I did one example with them and took questions. Then they were on their way.
Since they sit at tables, and I didn't want to do different colors of sheets of paper (though I guess I could have), I had them draw dots in different colored pens (one per set) at the bottom right corner of their cards to avoid mix up.
Once they got as many pairs as they could, and I mentioned that depending on a variety of things they may not get pairs. Then I said they could try to find a "triple" pair for their remaining lone cards. After some time for this, then I asked if they can "pair up" the pairs to get a set of four .... Ultimately, I then said, or they noticed the "squiggle" cards, and we got to the point that there are FOUR separate problems, that each start with a squiggle card, so see if you can set up 4 different strings of "one steppers".
During all this they had their "notes" on pink paper that they were going to eventually tape in their notebooks.
This all took about an hour or so to give them think time, and not everyone was successful with all of them, but we came together as a class and talked it out and got the 4 sets of problems, and celebrated any successes they had. Some problems had 7 steps, some 6, some 4. Then students taped the notes in their books, and we copied down 2 of the problems into their notes with "reasons". Hopefully, this will aid in their homework tonight where they have to do a string of one steps for each problem.



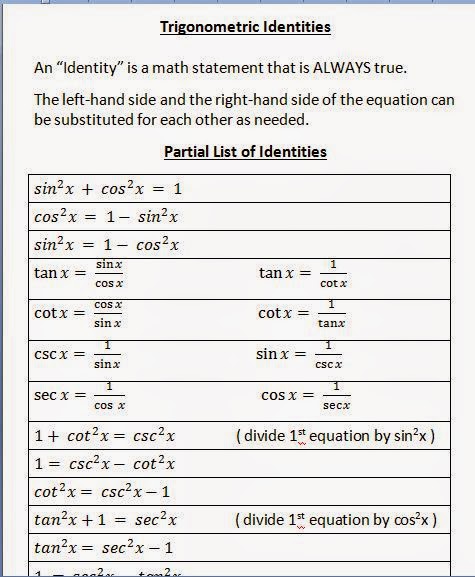
I really like this idea, especially having them figuring out for themselves that they had all the steps to the problems in front of them from the start. I feel this is a great way to get the students to work with each other and to recognize the different parts of the process of figuring out this type of problem.
ReplyDeleteKatie Jester's EDM 310 Blog
Shireen, what did you have them do on the previous day to explore?
ReplyDeleteMy Kids enjoyed this activity
ReplyDeleteI can't wait to try this with my class.
ReplyDeleteI can't wait to try this with my class.
ReplyDeleteI like this. I've done this with geometry proofs on notecards but for some reason didn't occur to me to try this with trig identities!
ReplyDeleteHi thanks for postinng this
ReplyDeleteThis sounds like an engaging way to help students learn trigonometric identities.
ReplyDelete